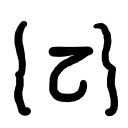Topologia e conjuntos em exercícios

Essa é uma revisão anterior do documento!

---

Para $\mathcal V$ ser um sistema de vizinhanças é preciso que seja um filtro sobre X e para todo $V \in \mathcal V$, $x \in V$.

$x \in V$ para todo $V \in \mathcal V$ pela própria definição de $\mathcal V$. E para ser filtro sobre X $\mathcal V$ precisa satisfazer as seguintes condições:

1. $\emptyset \notin \mathcal V$;
2. se $A, B \in \mathcal V$ então $A \cap B \in \mathcal V$;
3. se $A \in \mathcal V$ e $A \subset B$, então $B \in \mathcal V$.

Se $V \in \mathcal V$, $x \in V$ então $\emptyset \notin \mathcal V$.

Se $A, B \in \mathcal V$, $x \in A$ e $x \in B$ então $x \in A \cap B$. Como $A \subset X$ e $B \subset X$, então $A \cap B \subset X$. Logo, $A \cap B \in \mathcal V$.

Se $A \in \mathcal V$, então $x \in A$, e como $A \subset B$, $x \in B$. Portanto, $B \in \mathcal V$.

Como $\mathcal V$ também satisfaz as condições de filtro, $\mathcal V$ é um sistema de vizinhanças de $x$.